Chord construction

Audio: chord construction (0:14)

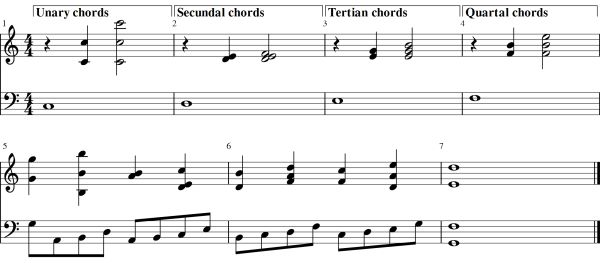
chord construction is a string quartet playing four part harmony in the key of C using chords constructed from different intervals. The chord construction figure shows the score:

• Bar 1: unary chords constructed from octaves.
• Bar 2: secundal chords constructed from seconds.
• Bar 3: tertian chords constructed from thirds.
• Bar 4: quartal chords constructed from fourths.
• Bars 5-6: a harmony written with a mix of these chords.
• Bar 7: a four note secundal chord, DEFG.

A chord is constructed from a root note upwards in pitch in defined intervals using the notes in a scale.

There are four possible intervals that can be used to construct a chord. Four, not eight, because intervals always come in pairs and what is true for an interval is true for its inversion. The four intervals are unison, second, third and fourth. They constitute unary, secundal, tertian and quartal harmony respectively.

A moment of reflection shows that a unary system of harmony is pretty much a nonstarter for constructing chords. Unary harmony is based on the interval of unison. A unary chord will only contain unisons and octaves. There will not be a lot of variety in unary harmony, to put it mildly. This leaves a choice between three systems:

1. Secundal harmony constructs a chord using the interval of a second:
   • A tone cluster is an example of a secundal chord.
   • Secundal harmony is rare largely because the interval of a second is widely regarded as a dissonant sound.
2. Tertian harmony constructs a chord using the interval of a third:
   • A triad is an example of a tertian chord.
   • Tertian harmony is the dominant system of harmony throughout the world and has been for the past few hundred years or so. It is such a dominant system that many books and websites on harmony assume the reader knows this already. Perhaps you did.
3. Quartal harmony constructs a chord using the interval of a fourth:
   • Quartal harmony was the dominant system of harmony many centuries ago and has been largely replaced, although not entirely, by tertian harmony.

Chord construction also enables us to determine whether a chord is in accord or not, in other words, whether it is a coherent or logical or structured entity.

We have only to look at a tuning system to be able to justify the use of intervals to derive the notes used in scales. The intervals from which scales are derived are the same intervals used to construct chords. Combining a tuning system with a logical system for constructing chords, such as tertian harmony, enables us to answer in the affirmative, yes, a chord is in accord because it is a coherent, logical and structured entity.

chord construction illustrates the logical nature of chord construction. The chords in bars 1-4 are constructed in unary, secundal, tertian and quartal harmony respectively using the notes in the major scale in the key of C. The root in the lower part of bar 1 is C and forms unary chords. The root in bar 2 is D and forms the secundal chords DE and DEF. Bar 3 forms the tertian chords EG and EGB, and bar 4 the quartal chords FB and FBE.

Tertian harmony is the dominant system in contemporary harmony. The theory is well-developed and the system is thoroughly documented. It underpins functional harmony and is widely used in pop harmony and jazz harmony. Most of the harmony in the guide is written using tertian harmony. The other systems, though much less well documented or understood, are also worth a look. A secundal chord, for example, is used in jazz harmony and chromatic harmony. A quartal chord is used in pop harmony and jazz harmony. As a music writer you are free, of course, to construct any type of chord in whatever manner you choose whenever you wish.